In the attached figure, you can see a chessboard and two rooks placed on the chess board. What you have to find is the number of squares that do not contain the rooks.How many are there?
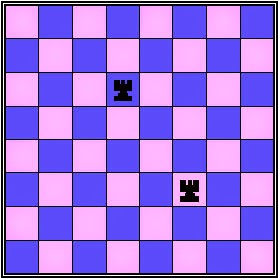
128
Lets count them one by one.
62 squares of size 1x1.
41 squares of size 2x2.
18 squares of size 3x3.
6 squares of size 4x4.
1 square of size 5x5.
62 + 41 + 18 + 6 + 1 = 128 squares.



